| comments | difficulty | edit_url | rating | source | tags | |||||
|---|---|---|---|---|---|---|---|---|---|---|
true |
Medium |
1885 |
Biweekly Contest 1 Q3 |
|
On a campus represented as a 2D grid, there are n workers and m bikes, with n <= m. Each worker and bike is a 2D coordinate on this grid.
We assign one unique bike to each worker so that the sum of the Manhattan distances between each worker and their assigned bike is minimized.
Return the minimum possible sum of Manhattan distances between each worker and their assigned bike.
The Manhattan distance between two points p1 and p2 is Manhattan(p1, p2) = |p1.x - p2.x| + |p1.y - p2.y|.
Example 1:
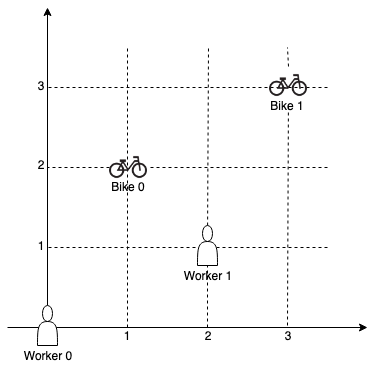
Input: workers = [[0,0],[2,1]], bikes = [[1,2],[3,3]] Output: 6 Explanation: We assign bike 0 to worker 0, bike 1 to worker 1. The Manhattan distance of both assignments is 3, so the output is 6.
Example 2:
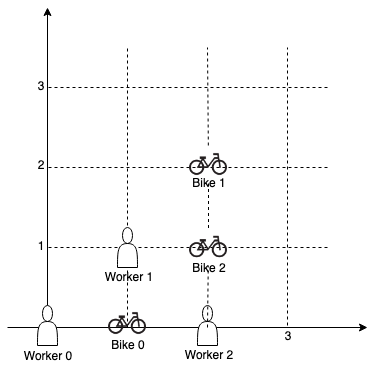
Input: workers = [[0,0],[1,1],[2,0]], bikes = [[1,0],[2,2],[2,1]] Output: 4 Explanation: We first assign bike 0 to worker 0, then assign bike 1 to worker 1 or worker 2, bike 2 to worker 2 or worker 1. Both assignments lead to sum of the Manhattan distances as 4.
Example 3:
Input: workers = [[0,0],[1,0],[2,0],[3,0],[4,0]], bikes = [[0,999],[1,999],[2,999],[3,999],[4,999]] Output: 4995
Constraints:
n == workers.lengthm == bikes.length1 <= n <= m <= 10workers[i].length == 2bikes[i].length == 20 <= workers[i][0], workers[i][1], bikes[i][0], bikes[i][1] < 1000- All the workers and the bikes locations are unique.
class Solution:
def assignBikes(self, workers: List[List[int]], bikes: List[List[int]]) -> int:
n, m = len(workers), len(bikes)
f = [[inf] * (1 << m) for _ in range(n + 1)]
f[0][0] = 0
for i, (x1, y1) in enumerate(workers, 1):
for j in range(1 << m):
for k, (x2, y2) in enumerate(bikes):
if j >> k & 1:
f[i][j] = min(
f[i][j],
f[i - 1][j ^ (1 << k)] + abs(x1 - x2) + abs(y1 - y2),
)
return min(f[n])class Solution {
public int assignBikes(int[][] workers, int[][] bikes) {
int n = workers.length;
int m = bikes.length;
int[][] f = new int[n + 1][1 << m];
for (var g : f) {
Arrays.fill(g, 1 << 30);
}
f[0][0] = 0;
for (int i = 1; i <= n; ++i) {
for (int j = 0; j < 1 << m; ++j) {
for (int k = 0; k < m; ++k) {
if ((j >> k & 1) == 1) {
int d = Math.abs(workers[i - 1][0] - bikes[k][0])
+ Math.abs(workers[i - 1][1] - bikes[k][1]);
f[i][j] = Math.min(f[i][j], f[i - 1][j ^ (1 << k)] + d);
}
}
}
}
return Arrays.stream(f[n]).min().getAsInt();
}
}class Solution {
public:
int assignBikes(vector<vector<int>>& workers, vector<vector<int>>& bikes) {
int n = workers.size(), m = bikes.size();
int f[n + 1][1 << m];
memset(f, 0x3f, sizeof(f));
f[0][0] = 0;
for (int i = 1; i <= n; ++i) {
for (int j = 0; j < 1 << m; ++j) {
for (int k = 0; k < m; ++k) {
if (j >> k & 1) {
int d = abs(workers[i - 1][0] - bikes[k][0]) + abs(workers[i - 1][1] - bikes[k][1]);
f[i][j] = min(f[i][j], f[i - 1][j ^ (1 << k)] + d);
}
}
}
}
return *min_element(f[n], f[n] + (1 << m));
}
};func assignBikes(workers [][]int, bikes [][]int) int {
n, m := len(workers), len(bikes)
f := make([][]int, n+1)
const inf = 1 << 30
for i := range f {
f[i] = make([]int, 1<<m)
for j := range f[i] {
f[i][j] = inf
}
}
f[0][0] = 0
for i := 1; i <= n; i++ {
for j := 0; j < 1<<m; j++ {
for k := 0; k < m; k++ {
if j>>k&1 == 1 {
d := abs(workers[i-1][0]-bikes[k][0]) + abs(workers[i-1][1]-bikes[k][1])
f[i][j] = min(f[i][j], f[i-1][j^(1<<k)]+d)
}
}
}
}
return slices.Min(f[n])
}
func abs(x int) int {
if x < 0 {
return -x
}
return x
}function assignBikes(workers: number[][], bikes: number[][]): number {
const n = workers.length;
const m = bikes.length;
const inf = 1 << 30;
const f: number[][] = new Array(n + 1).fill(0).map(() => new Array(1 << m).fill(inf));
f[0][0] = 0;
for (let i = 1; i <= n; ++i) {
for (let j = 0; j < 1 << m; ++j) {
for (let k = 0; k < m; ++k) {
if (((j >> k) & 1) === 1) {
const d =
Math.abs(workers[i - 1][0] - bikes[k][0]) +
Math.abs(workers[i - 1][1] - bikes[k][1]);
f[i][j] = Math.min(f[i][j], f[i - 1][j ^ (1 << k)] + d);
}
}
}
}
return Math.min(...f[n]);
}